Website owner: James Miller
The Complex Inversion Formula. Bromwich contour.
The Complex Inversion Formula. If L[F(t)] = f(s), then
![]()
Evaluating this formula provides a direct means for obtaining the inverse Laplace transform of a given function f(s). The primary device used in evaluating it is the Method of Residues of Complex Variable theory.
The integration in 1) is performed along the vertical line s = α in the complex plane where s = x + iy. The real number α is chosen so that the line s = α lies to the right of all singularities (branch points, poles, essential singularities, etc.). Otherwise the line is arbitrary.
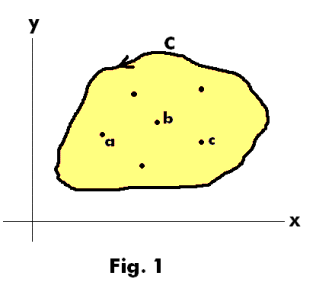
The Method of Residues provides a means of computing the values of complex line integrals taken around simple closed curves in the complex plane. The technique is based on the Residue theorem:
Residue theorem. Let f(z) be analytic inside and on a simple closed curve C except at the isolated singularities a, b, c, ... inside C which have residues given by ar, br, cr ... . Then
![]()
See Fig. 1.
For information on methods and procedures for calculating the residues at the various singular points see Method of Residues.
The procedure followed in evaluating formula 1) above depends on whether the function f(s) does or does not contain branch points. We first consider the case in which f(s) contains no branch points.
Case 1. No branch points.
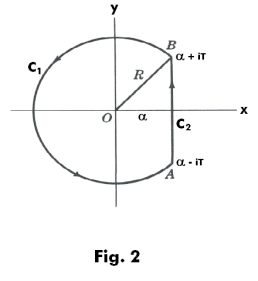
The Bromwich contour. The simple closed curve about which the integration is performed in evaluating formula 1) above is shown in Fig. 2 and is called the Bromwich contour. The curve C consists of two parts, C1 and C2, as shown in the figure. C1 is the portion of a circle of radius R, centered at the origin, shown in the figure. C2 is the vertical line AB located at a distance α to the right of the origin. Integration takes place in the counterclockwise direction on a limiting case of the curve shown in which the radius R is allowed to approach infinity. The integration corresponding to formula 1) takes place along the C2 portion of the curve. According to the residue theorem
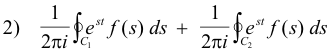
= sum of residues of all isolated singular points inside C
As R → ∞, the curve C will encompass all isolated singular points. In Fig. 2, points A and B have the complex coordinates α - iT and α + iT, respectively, where
![]()
Thus 2) becomes
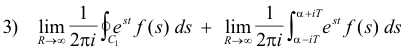
= sum of residues of all isolated singular points inside C
Now in certain circumstances the integral along curve C1 approaches zero as R → ∞. In such cases the integral along curve C2 ( i.e. the complex inversion integral) is equal to the sum of the residues of all isolated singular points inside C. The circumstances under which the integral along curve C1 approaches zero as R → ∞ is given by the following theorem:
Theorem 1. If we can find constants M > 0, k > 0 such that on C1 (where s = Reiθ),
![]()
then the integral along C1 of est f(s) approaches zero as R → ∞, i.e.
![]()
It can be shown that the condition 4) always holds if f(s) = P(s)/Q(s) where P(s) and Q(s) are polynomials and the degree of P(s) is less than the degree of Q(s).
Example 1. Evaluate
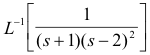
by the method of residues.
Solution.
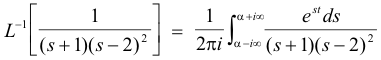
The integrand has a simple pole at s = -1 and a double pole at s = 2. In addition, condition 4) of Theorem 1 is satisfied since the function is a quotient of two polynomials in which the degree of the numerator is less than that of the denominator. Consequently the integral along curve C1 of Fig. 2 is zero and the integral along C2 (the complex inversion integral) is equal to the sum of the residues of the two poles.
The residue at the simple pole s = -1 is
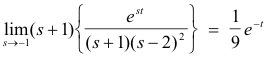
The residue at the double pole s = 2 is
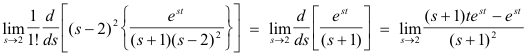
![]()
Thus
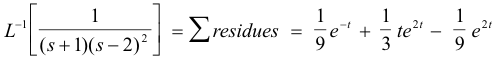
Case 2. Branch points.
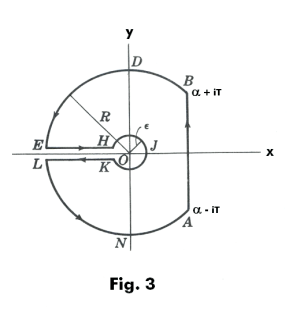
Modification of the Bromwich contour in case of branch points. The above procedure was based on the assumption that the function f(s) contained no branch points. If the function f(s) does contain branch points, a modification of the Bromwich contour must be made. In essence, a path of integration is then chosen that excludes the branch points. If, for example, f(s) has only one branch point located at s = 0, then we can use the contour shown in Fig. 3. In this case integrals along paths BDE and LNA will be zero if Theorem 1 is satisfied. However, integrals along paths EH, HJK, and KL will, in general, be non-zero and the integral about the entire contour will, by the residue theorem, be equal to the sum of the residues of all isolated singular points (poles, etc.) enclosed by the contour. By computing the residues and the integrals along paths EH, HJK, and KL we can obtain the value of the integral along path AB (i.e. the value of the complex inversion integral) by
![]()
Example 2. Find
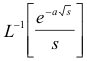
by the complex inversion formula.
Solution. By the complex inversion formula, the required inverse Laplace transform is given by
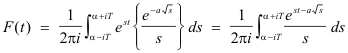
Here the integrand contains a branch point at s = 0. It has no isolated singular points. We will
use the Bromwich contour shown in Fig. 3. The integrand satisfies the conditions of Theorem 1
so that on taking the limit as R
![]() the integrals along BDE and LNA approach zero. Thus
the integrals along BDE and LNA approach zero. Thus
![]()
so
![]()
We now evaluate the integrals along the paths EH, KL, and HJK.
Along EH,
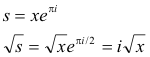
and as s goes from -R to -ε, x goes from R to ε. Thus

Along KL,
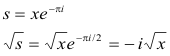
and as s goes from -ε to -R, x goes from ε to R. Thus
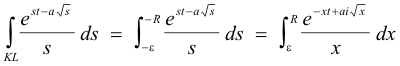
Along HJK,
s = εeiθ
and
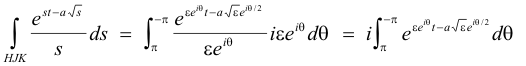
We thus obtain

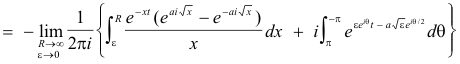

Since the limit can be taken underneath the integral sign, we have
![]()
and so we obtain
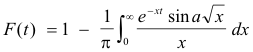
This can be written as
![]()
References
Murray R. Spiegel. Laplace Transforms. (Schaum)
Jesus Christ and His Teachings
Way of enlightenment, wisdom, and understanding
America, a corrupt, depraved, shameless country
On integrity and the lack of it
The test of a person's Christianity is what he is
Ninety five percent of the problems that most people have come from personal foolishness
Liberalism, socialism and the modern welfare state
The desire to harm, a motivation for conduct
On Self-sufficient Country Living, Homesteading
Topically Arranged Proverbs, Precepts, Quotations. Common Sayings. Poor Richard's Almanac.
Theory on the Formation of Character
People are like radio tuners --- they pick out and listen to one wavelength and ignore the rest
Cause of Character Traits --- According to Aristotle
We are what we eat --- living under the discipline of a diet
Avoiding problems and trouble in life
Role of habit in formation of character
Personal attributes of the true Christian
What determines a person's character?
Love of God and love of virtue are closely united
Intellectual disparities among people and the power in good habits
Tools of Satan. Tactics and Tricks used by the Devil.
The Natural Way -- The Unnatural Way
Wisdom, Reason and Virtue are closely related
Knowledge is one thing, wisdom is another
My views on Christianity in America
The most important thing in life is understanding
We are all examples --- for good or for bad
Television --- spiritual poison
The Prime Mover that decides "What We Are"
Where do our outlooks, attitudes and values come from?
Sin is serious business. The punishment for it is real. Hell is real.
Self-imposed discipline and regimentation
Achieving happiness in life --- a matter of the right strategies
Self-control, self-restraint, self-discipline basic to so much in life