Quadratic forms, canonical forms of conics with centers

SolitaryRoad.com

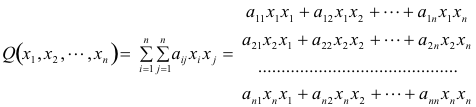
Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

QUADRATIC FORMS, CANONICAL FORMS OF CONICS WITH CENTERS

Quadratic form. A quadratic form is a homogeneous polynomial of degree two. The following are quadratic forms in one, two and three variables:

F(x) = ax²

F(x,y) = ax² + by² + cxy

F(x,y,z) = ax² + by² + cz² + dxy + exz + fyz

The polynomial consists of squared terms for each of the variables plus cross-products terms for all combinations of the variables.

Quadratic forms occur in many branches of mathematics and its applications. They are encountered in the theory of numbers, in crystallography, in the study of surfaces in analytic geometry, and in various problems of physics and mechanics.

It is customary to represent the quadratic form as a symmetric bilinear form

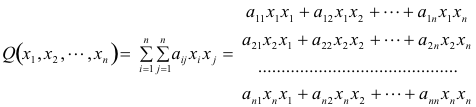
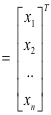
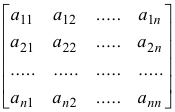
= X^TAX

where A is a symmetric matrix of the coefficients. Note that in this representation each cross-product term appears twice. For example, the x₁x₂ term appears as both an x₁x₂ term and a x₂x₁ term. Because we want the matrix to be symmetric we allocate half of the value of the coefficient to each term. The motivation behind representing the quadratic form in this manner is presumably simplification of formulas and to facilitate analysis and treatment.

The matrix A is called the matrix of the quadratic form and the rank of A is called the rank of the form. If the rank is less than n the quadratic form is called singular. If the rank is equal to n it is nonsingular.

Application in plane analytic geometry. In plane analytic geometry we are interested in the quadratic form

1) F(x, y) = ax² + 2hxy + by²

which can be written as

or in matrix form as

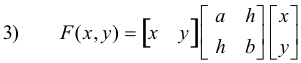
What is the importance of this quadratic form F(x, y)? Of what relevance is it in connection with investigations of the general equation of the second degree

4) f(x, y) = ax² + 2hxy + by² + 2gx + 2fy + c = 0 ?

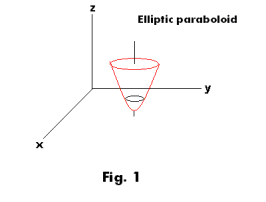
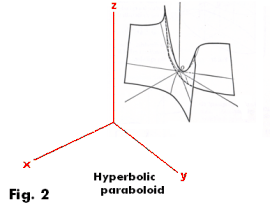
Let us consider the function z = f(x, y) associated with the equation f(x, y) = 0. The function z = f(x, y) is a paraboloid (either an elliptic or hyperbolic paraboloid) which is symmetric about some vertical axis located at the conic center. See figures 1 and 2. Let us assume that 4) represents a central conic. In this case we can eliminate the x and y terms in z = f(x, y) by translating the coordinate system to the center (x₀, y₀) of the conic.

If we translate the coordinate system the function f(x, y) will become

6) g(x', y') = ax'² + 2hx'y' + by'² + c'

where

c' = gx₀ + fy₀ + c .

Then in the x'- y' system g(x', y') is given by the expression

g(x', y') = F(x', y') + c' .

(where we have simply replaced the variable names x, y in the function F(x, y) with x', y').

The function g(x', y') is the same surface as F(x, y) but at a different distance above the xy-plane than F(x, y) — the vertex of F(x, y) touches the x-y plane at the origin and the vertex of g(x', y') is at a distance c' above it. If we do a suitable rotation on F(x, y) we can eliminate the xy term and reduce it to canonical form. The same rotation will eliminate the xy term of g(x', y'). A rotation represents a rotation of F(x, y) about the vertical axis.

A theorem of matrix theory states the following:

Theorem 1. Every real quadratic form q = X^TAX with symmetric matrix A can be reduced by an orthogonal transformation X = BY to a canonical form

λ₁y₁² + λ₂y₂² + ... + λ_ny_n² ,

where λ₁, λ₂, ... ,λ_n are the characteristic roots of A.

We can restate this theorem for our particular case as follows:

Theorem 2. By a suitable rotation of axes the quadric surface

F(x, y) = ax² + 2hxy + by²

is reducible to the form

λ₁x² + λ₂y²

where λ₁, λ₂ are the characteristic roots (i.e. eigenvalues) of the matrix

What does this theorem mean for us? This theorem gives us the canonical form of those conics that have centers.

Canonical form of conics with centers. Let

f(x, y) = ax² + 2hxy + by² + 2gx + 2fy + c = 0

be a central conic (ellipse or hyperbola). Let λ₁, λ₂ be the characteristic roots of matrix

and let (x₀, y₀) be a center. The canonical form of the conic is

7) λ₁x² + λ₂y² + c' = 0

where

c' = gx₀ + fy₀ + c