Functions, mappings, operators, transformations

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

FUNCTIONS, MAPPINGS, OPERATORS

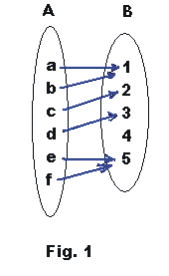
Def. Function (or mapping). An assignment of exactly one object from one set (the co-domain) to each object of another set (the domain). See Fig. 1. A is the domain and B is the co-domain. To each element of set A is assigned an element of set B.

Syn. operator, transformation

In this very abstract, general definition the elements (or objects) of set A can be anything. Likewise for set B. The “objects” of set A might be integers, real numbers, complex numbers, points in n-space, vectors, matrices, functions, etc. Likewise for set B.

Example. We can say that a person’s age is a function of the person. Here set A is the set of all human beings and set B is the set of all integers between, say, 1 and 150.

How we think of a function or mapping depends on the situation. Consider the function

1) u = f(x, y, z)

where x, y, z and u are real numbers. We think of this function as assigning a value (some real number) to each point (x, y, z) of 3-space. Likewise, the function,

2) u = f(x₁, x₂, ... ,x_n)

where x₁, x₂, ... ,x_n and u are real numbers, is viewed as assigning some real number to each point (x₁, x₂, ... ,x_n) of n-space. Now consider the mapping represented by the system

3) u = u(x, y)

v = v(x, y)

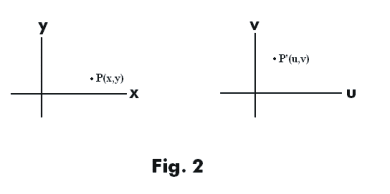
where x, y, u, v are real numbers. Here the number pair (x, y) is mapped into the number pair (u, v) — said differently, number pair (u, v) is assigned to number pair (x, y). To clearly envision this we utilize two coordinate systems — an x-y coordinate system and a u-v coordinate system. See Fig. 2. Point

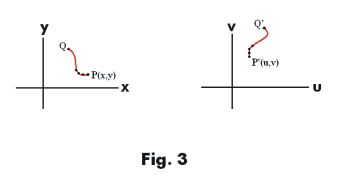
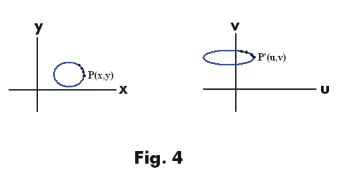
P(x, y) corresponds to a point in the x-y coordinate system and point P'(u, v) corresponds to the image of P(x, y) in the u-v coordinate system. Transformation 3) can be viewed as an operator that operates on the number pair (x, y) to produce the number pair (u, v). That is, it can be viewed as a black box with an input and an output. The input is point (x, y). The output is point (u, v). We can view point (x, y) as mapped into point (u, v) or we can view point (u, v) as being assigned to point (x, y), whichever we prefer. Both amount to the same thing. To better understand how the transformation behaves we ask ourselves what some path of points in the x-y system maps into in the u-v system — or what some figure, such as a circle, in the x-y system maps into in the u-v system. See Fig. 3 and 4. The transformation effected by 3) is also called a point transformation. A point in the x-y system is transformed into a point in the u-v system.

In a similar way the system

4) u = u(x, y, z)

v = v(x, y, z)

w = w(x, y, z)

where x, y, z, u, v, w are real numbers can be viewed as a point transformation (or mapping) from 3-space into 3-space and the system

5) u₁ = u₁(x₁, x₂, ... ,x_n)

u₂ = u₂(x₁, x₂, ... ,x_n)

..............................

u_n = u_n(x₁, x₂, ... ,x_n)

where x₁, x₂, ... ,x_n and u₁, u₂, ... ,u_n are real numbers can be viewed as a point transformation or mapping from n-space into n-space. The system

6) x = x(t)

y = y(t)

z = z(t)

where t, x, y, z are real numbers represents a mapping from 1-space into 3-space and the system

7) x = x(u, v)

y = y(u, v)

z = z(u, v)

where u, v, x, y, z are real numbers represents a mapping from 2-space into 3-space.

Let us now note something. Although the terms function, mapping, transformation and operator are all defined by the same definition and considered to be synonymous there tends to be a difference in how we think of them. We tend to think of a function as assigning a value to a point in n-space as in 1) and 2) above and to think of a mapping, operator, or point transformation as mapping a point in one coordinate system into another point in another coordinate system.