denoting the principal n-th root of a. The positive
integer n is the index, or order, of the radical and the number a is the radicand. The index is
omitted if n = 2.
Website owner: James Miller
Radicals. Laws. Simplification. Reduction of the index. Rationalization of the denominator.
Def. Radical. An expression of the form
denoting the principal n-th root of a. The positive
integer n is the index, or order, of the radical and the number a is the radicand. The index is
omitted if n = 2.
The laws for radicals are obtained directly from the laws for exponents by means of the definition
Laws of Radicals. If n is even, assume a, b ≥ 0.
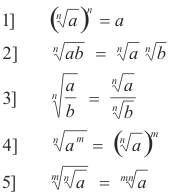
Simplification of radicals. It is important to reduce a radical to its simplest form through use of the following operations.
1. Removal of perfect n-th powers from a radicand. Any radical of order n should
be simplified by removing all perfect n-th powers from under the radical sign using the rule
.
Examples.
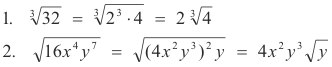
2. Reduction of the index of the radical.
Examples.
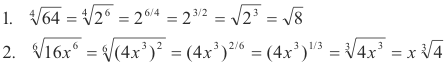
In the first example the index was reduced from 4 to 2 and in the second example it was reduced from 6 to 3. We note that the process involves converting to exponential notation and then converting back.
3. Rationalization of the denominator. Fractions may be removed from under a radical sign by rationalizing the denominator. To rationalize the denominator of a radical of order n, multiply the numerator and denominator of the radicand by such a quantity as will make the denominator a perfect n-th power and then remove the denominator from under the radical sign.
Examples.
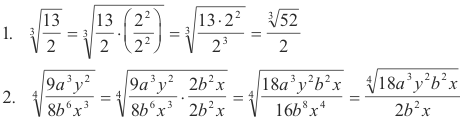
A radical is said to be in simplest form if
1) all perfect n-th powers have been removed from the radical
2) the index of the radical is as small as possible
3) no fractions are present in the radicand i.e. the denominator has been rationalized
Def. Similar radicals. Radicals which, on being reduced to simplest form, have the same index and radicand.
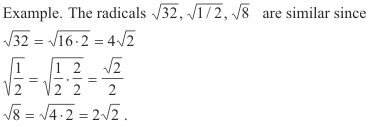
Addition and subtraction of radicals. Before addition or subtraction of radicals it is important to reduce them to simplest form. Like radicals can then be added or subtracted in the same way as other like terms.
Example.
Multiplication of radicals.
1) To multiply two or more radicals having the same index use
.
Examples.
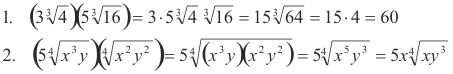
2) To multiply radicals with different indices use fractional exponents and the laws of exponents.
Example.
Division of radicals.
1) To divide two radicals having the same index use
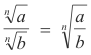
and simplify.
Example.
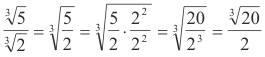
2) To divide radicals with different indices use fractional exponents and the laws of exponents.
Example.
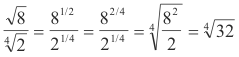
Rationalization of the denominator in fractions with binomial
irrational denominators. In one form of fraction, the denominator is a binomial in
which one term is a square root of a rational number or expression and the other term is of the
same form or is rational i.e. the denominator has the form
. In such a case,
rationalize the fraction by multiplying the numerator and denominator by the conjugate of the
denominator - where the conjugate of
and the conjugate of
.
Example.
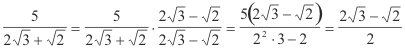
References
Hawks, Luby, Touton. Second-Year Algebra
Murray R. Spiegel. College Algebra
Raymond W. Brink. A First Year of College Mathematics
Jesus Christ and His Teachings
Way of enlightenment, wisdom, and understanding
America, a corrupt, depraved, shameless country
On integrity and the lack of it
The test of a person's Christianity is what he is
Ninety five percent of the problems that most people have come from personal foolishness
Liberalism, socialism and the modern welfare state
The desire to harm, a motivation for conduct
On Self-sufficient Country Living, Homesteading
Topically Arranged Proverbs, Precepts, Quotations. Common Sayings. Poor Richard's Almanac.
Theory on the Formation of Character
People are like radio tuners --- they pick out and listen to one wavelength and ignore the rest
Cause of Character Traits --- According to Aristotle
We are what we eat --- living under the discipline of a diet
Avoiding problems and trouble in life
Role of habit in formation of character
Personal attributes of the true Christian
What determines a person's character?
Love of God and love of virtue are closely united
Intellectual disparities among people and the power in good habits
Tools of Satan. Tactics and Tricks used by the Devil.
The Natural Way -- The Unnatural Way
Wisdom, Reason and Virtue are closely related
Knowledge is one thing, wisdom is another
My views on Christianity in America
The most important thing in life is understanding
We are all examples --- for good or for bad
Television --- spiritual poison
The Prime Mover that decides "What We Are"
Where do our outlooks, attitudes and values come from?
Sin is serious business. The punishment for it is real. Hell is real.
Self-imposed discipline and regimentation
Achieving happiness in life --- a matter of the right strategies
Self-control, self-restraint, self-discipline basic to so much in life