Double integral, triple integral, iterated integral

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

DOUBLE INTEGRAL, TRIPLE INTEGRAL, ITERATED INTEGRAL

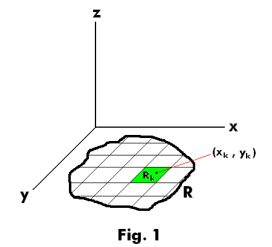
Def. Double integral. Let z = f(x, y) be a function defined and continuous over a finite region R of the xy-plane. Let this region be subdivided into n subregions R₁, R₂, ... ,R_n of respective areas ΔA₁, ΔA₂, ... ,ΔA_n. See Fig. 1. The manner of subdivision is arbitrary. In each subregion R_k we select a point P_k(x_k, y_k) and form the sum

Next we define the diameter r_i of a subregion R_i to be the greatest distance between any two points within or on its boundary. The double integral of the function f(x, y) over the region R is then defined as

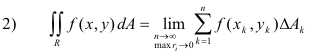
where we stipulate that the maximum r_i → 0 as n → 0.

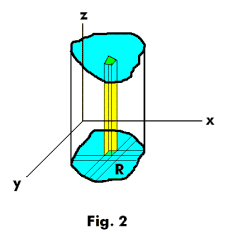
When z = f(x, y) is non-negative over the region R the double integral 2) may be interpreted as a volume. Any term f_k(x_k, y_k)ΔA_k of 1) gives the volume of a vertical column standing on ΔA_k as a base and of height z_k. See Fig. 2. The sum of all these terms gives an approximation to the volume of the cylindrical column standing on a base consisting of the region R, sides generated by a line parallel to the z axis moving along the boundary of R, and top, the cut-off section of surface z = f(x, y).

Thus we see that in exact analogy to the way we defined the definite integral

as the limit of a sum and interpreted it as an area, we define the double integral as the limit of a sum and interpret it as a volume.

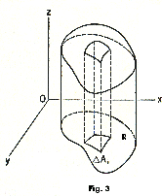
The iterated integral. Consider the function z = f(x, y) defined over a region R as shown in Fig. 3. The double integral of this function over region R is defined by 2) above. We will now demonstrate a method for computing this integral. We know how to find the volume of various solids by the method of slicing. The volume is expressed as the integral

where A(x) represents the area of a variable cross-section of the solid, all the sections being made by planes perpendicular to a fixed line. We shall now employ this method to evaluate the double integral.

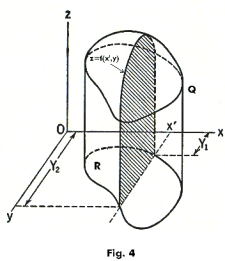
Consider the cylindrical column shown in Fig. 4. Label the cylindrical column “Q”. Let a plane x = x', parallel to the yz-plane, be passed through column Q as shown. The cross-section of column Q created by this plane corresponds to the area under the curve z = f(x', y) between points (x', Y₁) and (x', Y₂), where (x', Y₁) and (x', Y₂) are the points where the intersecting plane crosses the boundary of region R. The area of this cross-sectional slice is given by the integral

Consider the drawing of region R shown in Fig. 5. Let a be the smallest value which x can have in R, and b the largest value. R is assumed to be a region such that the line x = x', for each value of x' between a and b, cuts the boundary of R just twice, no more. The corresponding values of y are Y₁ and Y₂, where Y₁ < Y₂. For a given value of x', the values of Y₁ and Y₂ can be found from the equations of the boundary of R where y = Y₁(x) defines one part of the boundary and y = Y₂(x) defines the other part.

Upon dropping the primes, 4) can be written as

The expression A_s(x) plays the role of A(x) in formula 3), representing a slice of cylinder Q. Hence the volume V of cylinder Q is given by the integral

The inner integration, with respect to y, is to be performed first. The result is a function of x, which is then integrated between the limits x = a and x = b. In the first integration x is regarded as a constant.

The expression 5) for V is called an iterated integral. It is usually written as

we have just shown the technique for evaluating double integrals. They are evaluated by an iterated integral. The relationship between iterated integrals and double integrals is so important that we state it in a theorem:

Theorem 1. The double integral of a continuous function f(x, y) over a region A of the plane can be evaluated by either of the two iterated integrals:

where the limits of integration are determined with reference to the boundary of area A.

Def. Iterated integral. An indicated succession of integrals in which integration is to be performed first with respect of one variable, the others being held constant, then with respect to a second, the remaining ones being held constant, etc.

Indefinite iterated integrals. The integral

is an indefinite iterated integral. It is interpreted as

where the inner integral, ∫ f(x, y) dy, is integrated first, with respect to y, regarding x as a constant, and then the outer integration is performed with respect to x, regarding y as a constant. Each integration thus performed is called partial integration, and is the inverse of partial differentiation.

Example 1. Find

Solution.

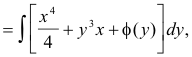
where the arbitrary function was introduced in order to provide the most general function whose partial derivative with respect to x is x³ + y³. Now performing the remaining integration with respect to y, we obtain

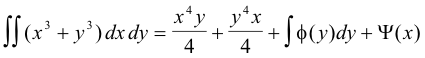
where Ψ(x) and Φ(y) are arbitrary functions.

One can confirm the same result will be obtained by reversing the order of integration.

The above integration is verified by observing that

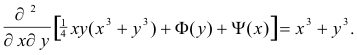
Definite iterated integrals. The integral

is a definite iterated integral where a and b are constants and u₁ and u₂ are either constants or functions of x. The inner integral is integrated first with respect to y, regarding x as constant, and then the outer integral is integrated with respect to x, regarding y as constant.

This notation can be extended to functions of any number of variables. Thus

represents a definite iterated integral in three variables.

Example 1. Evaluate

Solution.

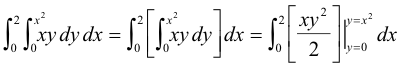
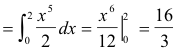
Area computation using double integrals.

Problem. Compute the area of PQRS bounded by the curves y = f(x) and y = φ(x) and the ordinates at x = a and x = b shown in Fig. 6 using double integrals.

Solution.

If we were to compute this area using single integrals we would get

Note that this integral is exactly the same as

Example. Compute the area enclosed by the parabola y² = 2x + 4 and the line y = x - 2. See Fig. 7.

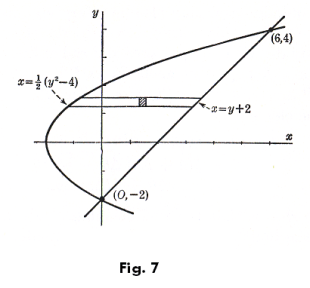
Solution. We will integrate first with respect to x, summing the little area elements in a horizontal strip extending from the parabola to the line. The limits of integration are from x = ½ (y² - 4) to x = y + 2. We then sum these strips by integrating with respect to y between the limits y = -2 and y = 4. Thus

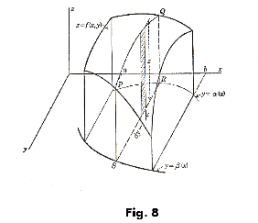
Volume computation by double integration.

Problem. Compute the volume between the xy-plane and the surface z = f(x, y), bounded on the sides by two arbitrary cylindrical surfaces y = α(x) and y = β(x), and the planes x = a and x = b, as shown in Fig. 8 using double integrals

Solution. The formula is

Example.

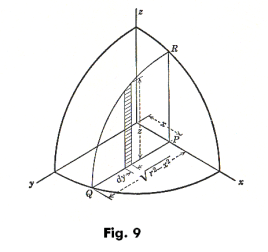
Express the volume of one octant of the sphere x² + y² + z² = r² as a double integral.

See Fig. 9.

Solution.

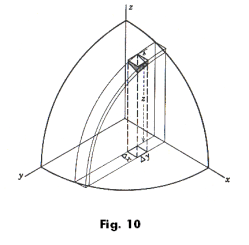
It is important to interpret this integration as a double summation process. The quantity z Δx Δy is the volume of a rectangular column with base Δy by Δx and height z, as indicated in Fig. 10. One can see that the first integration (with respect to y) sums these columns into a slice extending from y = 0 to y = The second integration, then, sums all the slices from x = 0 to x = r.

****************************

Triple integrals.

****************************

Def. Triple integral. Let f(x, y, z) be a function defined and continuous over a finite region R of xyz-space. Suppose that R is divided into n subregions R₁, R₂, ... ,R_n of volumes ΔV₁, ΔV_{2, .... ,}ΔV_n, respectively. In each subregion R_k we select a point P_k(x_k, y_k, z_k) and form the sum

Next we define the diameter r_i of a subregion R_i to be the greatest distance between any two points within or on its boundary. The triple integral of the function f(x, y) over the region R is then defined as