Electrochemistry. Ion. Electrolyte. Electrolysis. Electrolytic cell. Anode. Cathode. Ionic compound. Electroplating. Faraday�s laws of electrolysis. Electrode potential. Voltaic cell. Dry cell. Daniell cell. Gravity cell. Lead storage battery. Thermoelectricity. Seebeck effect. Peltier effect. Thomson effect.

SolitaryRoad.com

Website owner:  James Miller

[ Home ] [ Up ] [ Info ] [ Mail ]

Electrochemistry. Ion. Electrolyte. Electrolysis. Electrolytic cell. Anode. Cathode. Ionic compound. Electroplating. Faraday’s laws of electrolysis. Electrode potential. Voltaic cell. Dry cell. Daniell cell. Gravity cell. Lead storage battery. Thermoelectricity. Seebeck effect. Peltier effect. Thomson effect.

Michael Faraday observed that pure water was an almost total insulator, but that aqueous solutions of certain substances would conduct electricity. If we were to place two platinum electrodes in a beaker of pure (distilled) water and connect them to a battery and ammeter as shown in Fig. 1, the ammeter would show almost no current. If now we were to add a small amount of an acid such as sulfuric acid (H₂SO₄) or hydrochloric acid (HCl) to the water, we would see a fair sized current. The same is true if we were to add a small amount of a base such as sodium hydroxide (NaOH) or a salt such as common table salt (NaCl). Again, we would note an appreciable current (where the amount of current depends strongly on concentration and temperature). Aqueous solutions of many substances, however, do not conduct electricity. Solutions of most organic compounds, such as sugar, are nonconducting.

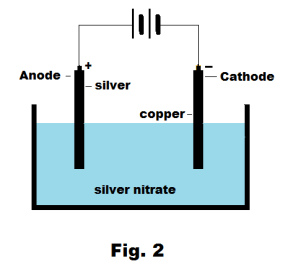
Let us suppose that we have added a small amount of sulfuric acid to the water in the beaker of Fig. 1 and that current is flowing. We would discover an interesting phenomenon. We would find that hydrogen gas was bubbling up around the negative electrode and oxygen was bubbling up around the positive electrode. Why would this happen? How do you account for it? Suppose now that we use a silver rod as the positive electrode and a copper rod as the negative electrode and replace the sulfuric acid solution with a solution of silver nitrate in water. See Fig. 2. We now turn on the current and find that as current passes, a coating of silver forms on the copper rod. What is going on here? How do you explain this phenomenon?

In an attempt to answer such questions, a Swedish chemist, Avante August Arrhenius, proposed some ideas which have, with modifications, become the modern theory of ionization — a theory in which charged particles called ions exist in such conducting solutions and move from one electrode to another under the influence of an electric field.

Def. Ion. An atom or group of atoms (i.e. a radical) which has gained or lost one or more outer shell (valence) electrons, giving it a net positive or negative electrical charge.

When hydrogen chloride (HCl) is added to water it breaks down into H⁺ and Cl^- ions according to

HCl→ H⁺ + Cl^-

Here the H⁺ ion corresponds to the hydrogen atom minus it sole electron. The ion thus has a net positive charge since it has one proton and no electrons. The Cl^- ion corresponds to the chlorine atom with an extra electron in its outer shell giving it a net negative charge. What has happened is that the chlorine atom, which normally has seven electrons in its outer shell, has stolen the hydrogen atom’s electron in order to complete its outer shell. Let us consider the case of sulfuric acid. When sulfuric acid (H₂SO₄) is added to water, it breaks down into H⁺ and SO₄⁼ ions according to

H₂SO₄→ 2H⁺ + SO₄⁼

It thus breaks up into three ions — two H+ ions and an SO₄⁼ ion. Here the group of atoms representing the sulfate radical, SO₄⁼ , has two extra electrons which it has stolen from the two hydrogen atoms, giving it a net negative charge of -2e.

Let us now define some terms:

Def. Electrolyte. A chemical compound that ionizes when dissolved or molten and thus produces an electrically conductive medium. This includes most acids, salts and bases.

Def. Electrolysis. The passage of an electric current through an electrolyte with subsequent migration of positively and negatively charged ions to the negative and positive electrodes and ensuing chemical changes.

Def. Electrolytic cell. A cell in which electrolysis occurs, consisting of an electrolyte through which current from an external source is passed, by a system of electrodes, in order to produce an electrochemical reaction.

Def. Anode. (1) The positive electrode of an electrolytic cell. See Fig. 2. (2) The negative terminal of a voltaic or primary cell.

Def. Cathode. (1) The negative electrode of an electrolytic cell. See Fig. 2. (2) The positive terminal of a voltaic or primary cell.

Def. Anion. Negatively charged ion.

Def. Cation. Positively charged ion.

Def. Ionic compound. An ionic compound is a compound formed by ions bonding together through electrostatic forces. Upon dissolving, it breaks apart into its individual ions. Ionic compounds are usually formed by a bonding of metal and nonmetal ions. Example: table salt, NaCl.

Properties of ionic compounds. Following are the properties shared by the ionic compounds. Notice that the properties of ionic compounds relate to how strongly the positive and negative ions attract each other in an ionic bond.

• Ionic compounds form crystals. Ionic compounds form crystal lattices rather than amorphous solids. Although molecular compounds form crystals, they frequently take other forms plus molecular crystals typically are softer than ionic crystals.

• Ionic compounds have high melting points and high boiling points. High temperatures are required to overcome the attraction between the positive and negative ions in ionic compounds. Therefore, a lot of energy is required to melt ionic compounds or cause them to boil.

• Ionic compounds have higher enthalpies of fusion and vaporization than molecular compounds. Just as ionic compounds have high melting and boiling points, they usually have enthalpies of fusion and vaporization that may be 10 to 100 times higher than those of most molecular compounds. The enthalpy of fusion is the heat required to melt a single mole of a solid under constant pressure. The enthalpy of vaporization is the heat required to vaporize one mole of a liquid compound under constant pressure.

• Ionic compounds are hard and brittle. Ionic crystals are hard because the positive and negative ions are strongly attracted to each other and difficult to separate.

• Ionic compounds conduct electricity when they are dissolved in water.

• Ionic solids are good insulators. Although they conduct in molten form or in aqueous solution, ionic solids do not conduct electricity very well because the ions are bound so tightly to each other.

Source: Anne Helmenstine, Ph.D. About.com

Motion of ions in an electrolyte. Under the influence of an electric field, positive ions migrate slowly toward the negative electrode, and negative ions migrate toward the positive electrode, but not necessarily with the same speed. Because of the difference in speeds of the two kinds of ions, the positive ions carry, in general, a different portion of the current from that carried by the negative ions. Ionic speeds are small, of the order of a few hundred-thousandths of a centimeter per hour.

The electrolysis of water. The electrolysis of water involves passing an electric current through a dilute aqueous solution of sulfuric acid using the setup shown in Fig. 1 above employing two platinum electrodes. Hydrogen gas is produced at the negative electrode (cathode) and oxygen at the positive electrode (anode).

Water ionizes very slightly to form hydrogen ions (H⁺) and hydroxide ions (OH^-). Sulfuric acid (H₂SO₄) breaks up into two hydrogen ions (H⁺) and one sulfate ion (SO₄⁼ ). So what happens? The negatively charged cathode attracts the positively charged hydrogen H⁺ ions and they migrate to it, receive their missing electrons, and bubble up through the solution as hydrogen gas. Four hydrogen ions take four electrons from the cathode to form four hydrogen atoms, or two hydrogen molecules:

4H⁺ + 4e^-→ 2H₂ (Cathode reaction)

The hydroxide OH^- ions and the sulfate SO₄⁼ ions migrate to the positive electrode. Here two things might happen: either the hydroxide OH^- ions or the sulfate SO₄⁼ ions might be discharged. Although the sulfate ions outnumber the hydroxide ions by far, less voltage is required to discharge the OH^- ions. Consequently it is these ions that are discharged. Four liberated hydroxide ions produce two molecules of water and one oxygen molecule.

4OH^-→ 4e^- + 2H₂O + O² (Anode reaction)

Electroplating metals. When electroplating, the metallic object to be plated is used as the cathode of the cell. The anode consists of the plating metal. The electrolyte consists of an aqueous solution of a salt of the metal to be plated — for example, copper sulfate when plating copper or silver nitrate in plating silver. Suppose we wish to plate the brass bar shown in Fig. 4 with silver. We use a silver anode and an aqueous solution of silver nitrate for an electrolyte.

Water ionizes slightly to form hydrogen ions (H⁺) and hydroxide ions (OH^-). Silver nitrate

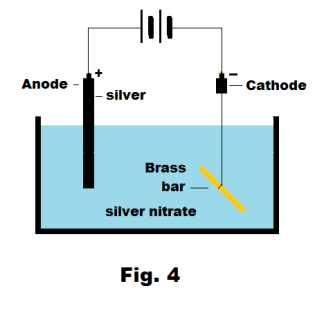
(AgNO₃) breaks up into silver ions (Ag⁺) and nitrate ions (NO₃^-). So what happens when the current is turned on? The negatively charged cathode attracts the positively charged hydrogen H⁺ ions and silver Ag⁺ ions and they migrate to it. The silver Ag⁺ ions are more easily discharged than the hydrogen H⁺ ions and so the Ag⁺ ions take on electrons and plate out as a layer of silver on the cathode:

Ag⁺ + e^- → Ag (Cathode reaction)

The hydroxide OH^- ions and the nitrate NO₃^-ions migrate to the positive electrode. Here, at the anode, three possible things can occur: 1) the OH^- ions can be discharged (give up their extra electron to the anode), 2) the NO₃^-ions can be discharged (give up their extra electron to the anode), 3) silver atoms in the anode can become silver ions according to

Ag - e^- → Ag⁺

This last option occurs at the lowest voltage of the three possible reactions and it is what occurs. The silver atoms give up an electron to the electrode and dissolve as Ag⁺ ions:

Ag - e^- → Ag⁺ (Anode reaction)

Hence, the anode is used up in the plating process and the process amounts to a transfer of silver from the anode to the cathode. The concentration of silver ions in the solution remains nearly constant. If the two electrodes are weighed before and after a plating session, it is found that the increase in weight of the cathode is matched exactly by the decrease in weight of the anode.

Faraday’s laws of electrolysis. Two laws of electrolysis stated by Faraday are:

(1) The mass of any substance liberated or deposited at an electrode is proportional to the total quantity of charge (in coulombs) passing through the electrolyte.

(2) If the same quantity of charge passes through a series of cells, the masses of the elements deposited or liberated are in the ratio of their respective equivalent weights.

Def. Equivalent weight of an element. The atomic weight of the element divided by the valence of its ion in the electrolyte.

Example. The equivalent weight of copper in an electrolyte containing the Cu⁺⁺ion is ½ its atomic weight or 63.6/2 = 31.8

Def. Gram-equivalent weight. The equivalent weight of a substance expressed in grams.

Def. Faraday. One faraday is the quantity of charge that will deposit 1 gram-equivalent weight of any substance.

The best experimental value of the faraday is 96,496 coul/gm atomic wt. Thus the mass m in grams of any substance liberated or deposited at an electrode is

m = gram-equivalent weight × number of faradays transferred

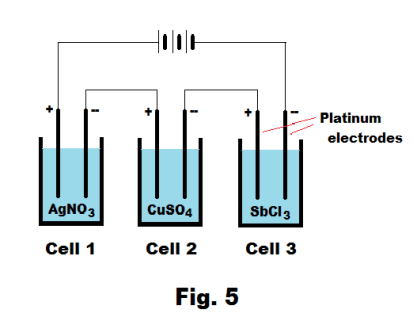
Consider the three electrolytic cells connected in series shown in Fig. 5. The electrolytes in them are aqueous solutions of AgNO₃, CuSO₄, and SbCl₃ as shown and all electrodes are platinum. Connecting them in series ensures that the current is the same in all cells. When current flows, silver will be deposited on negative electrode of Cell 1, copper on the negative electrode of Cell 2, and antimony on negative electrode of Cell 3. Let us allow current to flow for such a time as to allow one faraday, or 96,496 coulombs, of electricity to pass. Faraday’s law tells us that one gram-equivalent weight of each element should be deposited on the negative electrodes (i.e. cathodes). The metal ions in the electrolytes are Ag⁺, Cu⁺⁺, and Sb⁺⁺⁺. The gram-equivalent weights of the elements are

Silver: 108/1 = 108 gm

Copper: 63.6/2 = 31.8 gm

Antimony: 122/3 = 40.7 gm

Thus 108 gm of silver should be deposited on the cathode of Cell 1, 31.8 gm of copper should be deposited on the cathode of Cell 2, and 40.7 gm of antimony should be deposited on the cathode of Cell 3.

Def. Electrochemical equivalent of a substance. The number of grams of the substance liberated by one coulomb.

The mass m in grams of a substance liberated in electrolysis is

m = electrochemical equivalent × number of coulombs transferred

The determination of Avogadro’s number. Avogadro’s number N₀ is the number of molecules in one mole (i.e. one gram-molecular weight) of the substance. Faraday’s law can be used in conjunction with Millikan’s value for the electronic charge of a single electron to compute Avogadro’s number.

Let us consider the electrolysis of silver nitrate in which metallic silver is deposited on the cathode. Every silver ion that arrives at the negative electrode receives a single electronic charge (the charge of one electron) before it adheres as a neutral silver atom. If a mass of silver equal to its atomic weight is deposited, then N₀ silver atoms must have been transported. The total electric charge transferred is then N₀e, where e is the charge of an electron. But the total charge required to deposit one atomic weight is one faraday of electricity, F = 96,496 coulombs, so

1) N₀e = F

Using e = 1.6019 × 10^-19 coul/electron, we obtain

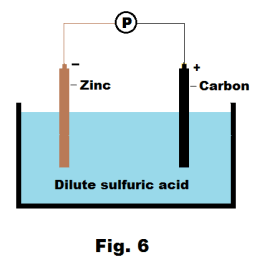
Electrode potential. If we place rods of two dissimilar conducting substances in a beaker of a conducting fluid (i.e. electrolyte), a potentiometer will show that a potential difference exists between them. In Fig. 6 the substances are zinc and carbon and the electrolyte is dilute sulfuric acid. How does one explain this phenomenon?

Let us consider what happens when a zinc rod is

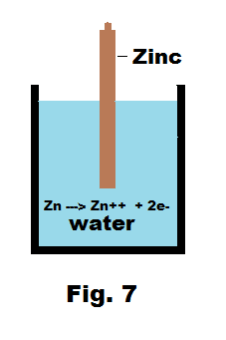
placed in a container of pure water as shown in Fig. 7. What occurs is that zinc atoms in the rod shed the two electrons in their outer shell and dissolve into the water as zinc Zn⁺⁺ions, leaving the electrons behind in the electrode

Zn→ Zn⁺⁺ + 2e^-

As this process proceeds, the zinc electrode builds up a negative charge with respect to the water. As the rod builds up a negative charge, a few of the Zn⁺⁺ ions are then attracted back to the negatively charged rod and neutralized. The greater the negative charge on the rod becomes, the more Zn⁺⁺ ions are attracted back and neutralized. These two processes go on in tandem and as the electrode becomes more and more negative they reach a point of equilibrium and the charge on the rod at the point of equilibrium represents the potential of the rod with respect to the water. This potential of the rod with respect to the water is called the electrode potential of the rod and its numerical value is of considerable importance in electrochemical processes. In general, the equilibrium potential of a substance with respect to a solution depends on both the temperature and the concentration of the dissolved ions near it.

The magnitude of the electrode potential of a metal at a standard temperature with a standard concentration of ions around it is a measure of the ease with which the metal will give up its outer shell (valence) electrons and go into solution. The greater the electrode potential, the greater the number of ions that leave the metal and go into solution. On the other hand, the electrode potential is also a measure of the ability (or inability) of an ion to discharge on an electrode and adhere to it. In this connection, it is often called the discharge potential. The greater the discharge potential, the greater is the inability of the ion to discharge on the electrode. For example, in the electrolysis of water, the discharge potential of the SO₄⁼ ion is so much larger than that of the OH^- ion that only the OH^- ion discharges. Similarly, in a solution of NaCl in water the discharge potential of the Na⁺ ion is so much larger than that of the H⁺ ion that only the H⁺ ion is discharged at the negative terminal.

How does one measure the electrode potential of a substance? One cannot measure the difference in potential between a substance and the solution it is immersed in. All one can do is

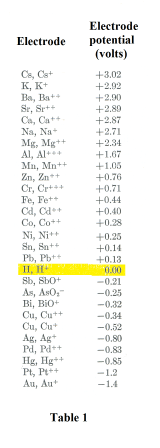
measure the difference in potential between two electrodes composed of two different substances immersed in a solution. So what do you do? You arbitrary pick a particular substance to use as a reference and then find the potentials of other substances relative to it i.e. you measure the potential differences existing between it and other substances for which you wish values. The reference substance that has been chosen is the normal hydrogen electrode which consists of a platinum rod that is kept saturated with hydrogen by bubbling hydrogen gas around it. It is immersed in an acid solution containing a standard concentration of H⁺ ions. Measurements are made, at a standard temperature of 25^o C, of the potential differences between it and electrodes of various substances surrounded by a standard concentration of their respective ions. See Fig. 8.

Table 1 shows the measured electrode potentials of various substances as referenced to the normal hydrogen electrode. Note that they are almost all metals. Also, note that hydrogen has the value 0. The potential difference between any two substances in the list is the difference between their values. For example, the

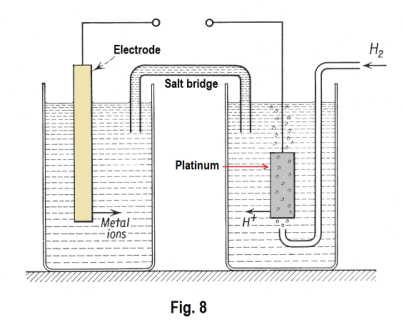
potential difference between zinc and copper is 0.76 - (-0.52) = 1.28. Roughly speaking, the metals at the top of the table tend to part with their ions easily, but their ions do not readily discharge on them. The metals at the bottom of the table do not easily send ions into solution, but their ions readily discharge on the electrodes.

The voltaic cell. A voltaic cell consists of two dissimilar conducting substances immersed in an electrolyte. Dozens of different types of voltaic cells have been devised. The metal most frequently used for the negative electrode is zinc. Many different substances have been used for the positive electrode, including carbon, copper, gold, silver, and platinum, with carbon being the one most commonly used. Various substances have been used as electrolytes in the various types of cells.

Working of a voltaic cell. Let us consider what occurs when current is flowing in a voltaic cell in which the negative electrode is zinc, the positive electrode is copper, and the electrolyte is a dilute solution of sulfuric acid (H₂SO₄). At the zinc electrode, the zinc goes into solution as zinc Zn⁺⁺ions while leaving its two valence electrons on the electrode, giving the electrode a negative charge:

Zn→ Zn⁺⁺ + 2e^-(Zinc electrode)

Besides the zinc Zn⁺⁺ions, the solution will also contain H+ ions from the slight ionization of water and the extensive ionization of the H₂SO₄ along with hydroxide OH^- ions and sulfate SO₄⁼ ions.

At the copper electrode, the hydrogen H+ ions discharge by taking electrons from the copper (thus giving the electrode a positive charge) and produce hydrogen gas according to

2H⁺ + 2e^- → H₂↑ (Copper electrode)

The hydrogen gas forms on the electrode and bubbles up.

As the process proceeds, the zinc electrode is used up. The zinc ions unite with the sulfate ions to form zinc sulfate ZnSO₄.

Defects of voltaic cells. Two common defects of voltaic cells are:

1. Local action. Carbon or coal is used in extracting zinc from its ores. Commercial zinc usually contains impurities in the form of particles of carbon distributed through it, left from the extraction process. These particles may become positively charged in the same way as the positive electrode, create simple electrolytic cells within the electrode, and cause it to wear away faster than if the zinc were pure. In addition, they may be set free as the zinc dissolves, become suspended in the electrolyte, and set up miniature circuits that result in wasted energy and degradation of cell function. Such chemical action is called local action. It can be decreased by a process called amalgamation involving rubbing the electrode with mercury.

2. Polarization. As a voltaic cell operates, the positive electrode tends to become coated with an accumulation of tiny bubbles of hydrogen gas. Thus an electrode of hydrogen has been effectively substituted for the carbon electrode we started with. The result is a gradual drop in voltage as the cell operates. The phenomenon is called polarization. To overcome this defect, manufacturers add a depolarizer such as potassium dichromate to the cell. The depolarizer unites chemically with the hydrogen and removes it from the carbon.

The dry cell. The dry cell is everywhere in our modern world, in many sizes and forms. An example is the ubiquitous flashlight battery. It is a very convenient small portable source of electric energy. It developed from the Leclanche cell invented by Georges Leclanche in 1868. The negative electrode is a zinc cylinder lined with porous cardboard. The positive electrode is a carbon rod, running down the center of the container, coated with a thin layer of manganese dioxide and powered carbon. Instead of a liquid electrolyte, the dry cell uses a paste consisting of ammonium chloride, zinc chloride, some inert filler, and a little water that occupies the space between the carbon rod and zinc shell. See Fig. 9. The top is covered with wax or asphalt to prevent water loss by evaporation.

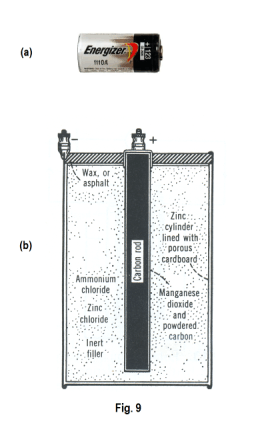
When the cell is operating, zinc atoms in the zinc shell electrode go into solution as zinc Zn⁺⁺ions. At the positive carbon electrode, ammonium ions gain electrons, forming ammonia gas and hydrogen. The ammonia gas is taken up by the zinc chloride. The hydrogen reacts with the manganese dioxide, which is present as a depolarizer.

A new dry cell has an open circuit emf of between 1.5 and 1.6 volts. Under long continuous use it may polarize, but recovers when allowed to stand on open circuit. Its internal resistance is so small that it may give a current of from 30 to 40 amperes. After extensive use, the internal resistance increases until the cell becomes useless.

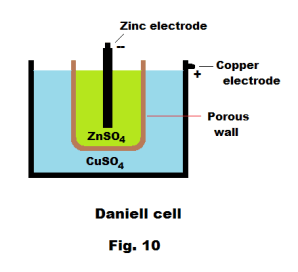
Daniell cell. The Daniell cell was invented in 1836 by John Frederic Daniell, a British chemist who was looking for a way to avoid the polarization problem of the voltaic cell. His solution was to use two different electrolytes contained in two different containers, one a copper container containing a solution of copper sulfate (CuSO₄) and, sitting inside it, another unglazed earthenware pot containing a solution of sulfuric acid (H₂SO₄). The walls of the pot serve to keep the solutions from mixing but are sufficiently porous to let ions pass back and forth. See Fig. 10. The original design was followed by several variations and this is one of the variations. One can substitute zinc sulfate (ZnSO₄) for the H₂SO₄ — which we have done. A zinc electrode immersed in the ZnSO₄ serves as the negative electrode. The positive electrode is the copper container. The copper sulfate solution contains Cu⁺⁺ ions and SO₄⁼ ions and the ZnSO₄ solution contains Zn⁺⁺ions and SO₄⁼ ions.

When the circuit is closed, the copper Cu⁺⁺ ions migrate to the positive copper electrode (i.e. the container wall) and are discharged, taking electrons from the electrode and depositing out on the electrode as copper atoms. At the negative electrode, zinc atoms give up two electrons to the electrode and dissolve into the solution as zinc Zn⁺⁺ ions. At the same time, zinc ions pass through the porous wall, combine with sulfate ions in the copper sulfate solution, and precipitate out as solid zinc sulfate. As current flows, the concentration of zinc sulfate increases, accompanied by precipitation of zinc sulfate, and the concentration of copper sulfate decreases. To correct this action, it is necessary to occasionally add water to dilute the zinc sulfate solution and to add copper crystals to replace the copper that deposits on the positive electrode. The net effect of the cell action is the disappearance of zinc from the zinc electrode and the appearance of copper on the positive electrode.

The Daniel cell produces around 1.1 volts and has a high internal resistance of about 2 ohms. Thus it produces currents of less than an ampere. It works best when operating continuously in a circuit of moderately high resistance. It was once used widely in the European telegraph industry and is now used mainly for classroom demonstrations.

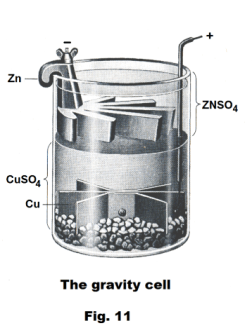
The gravity cell. The gravity cell is a variation of the Daniell cell invented by a Frenchman named Callaud in the 1860's. Shown in Fig. 11, it consists of a single glass container containing solutions of the two electrolytes ZnSO₄ and CuSO₄. There is no barrier between the electrolytes and they are kept separate by the fact that the zinc sulfate solution is less dense than the copper sulfate solution and by the polarity of the solutions. A layer of oil is often added to the top to prevent evaporation. A copper cathode sits on the bottom and a zinc anode is suspended beneath the rim in the zinc sulfate layer. The gravity cell quickly became the battery of choice for the American and British telegraph networks.

A disadvantage of the gravity cell is that a current has to be continually drawn to keep the two solutions from mixing by diffusion. Thus it is unsuitable for intermittent use. In addition, it is vulnerable to loss of integrity if too much electric current is drawn, which will also cause the layers to mix.

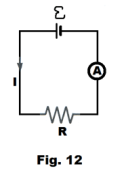
Measuring the internal resistance of a cell. To measure the internal resistance of a voltaic cell:

1. Determine the open circuit emf E of the cell using a potentiometer or a voltmeter.

2. Place the cell in a simple circuit containing a known resistance R and measure the amount of current I that flows with an ammeter. See Fig. 12.

3. Compute the internal resistance R_i of the cell from the equation

I = E /(R_i + R)

Factors affecting the performance of a cell. What factors affect the performance of a cell? Does the distance between the cell plates affect the performance? Does the size of the plates affect the performance? Does the amount of area of contact of the plates with the solution affect the performance?

Emf of a cell: The open circuit emf of a cell does not depend on the size of the plates, the distance between the plates, or the depth to which they are immersed in the solution. It is determined solely by the substances from which electrodes are made and the electrolyte used. Temperature has a slight effect.

Internal resistance of a cell: The internal resistance of a cell depends on the size of the plates, the distance between them, and the area of contact with the solution. To reduce internal resistance, use large plates and place them as close together as possible.

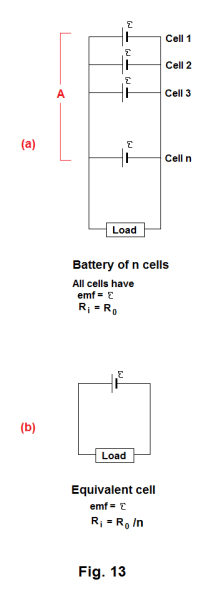
Cell equivalent to a battery of n parallel-wired cells. Let A represent a battery of n identical parallel-wired cells, all with emf E and internal resistance R₀, arranged in a circuit as shown in Fig. 13 (a). Then a single cell functionally equivalent to this battery A of n cells is a single cell of emf E with an internal resistance of R_i = R₀/n as shown in Fig. 13 (b).

Proof. Each of the cells in the battery has an internal resistance of R₀ and these resistances form a set of n parallel-wired resistances in the circuit as shown in Fig. 14. The equivalent resistance R_i of these n parallel-wired resistances is given by the formula

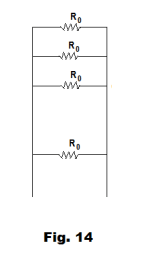
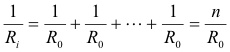
so R_i = R₀/n. Thus the equivalent cell has both the same emf and the same internal resistance as the n cell battery

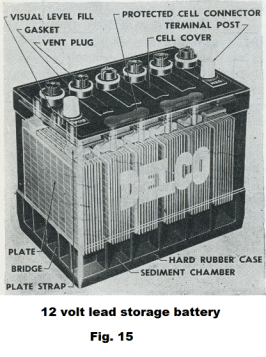
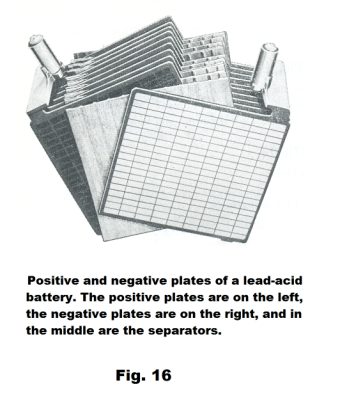
Lead storage battery. The lead-acid battery was invented in 1859 by French physicist Gaston Plante and is the oldest type of rechargeable battery. Fig. 15 shows the construction of a 12 volt lead storage battery. As can be seen in the picture, the battery contains six banks of cells where each bank consists of a set of n cells wired in parallel. Fig. 16 shows a single bank. Each cell of the bank consists of a positive plate, negative plate and a separator and has an emf of around 2.1 volts. Each bank delivers an emf of about 2.1 volts and the banks are wired in series to deliver a total voltage of around 12.6 volts.

Action at the plates. When a lead-acid battery is fully charged, the positive plates consist of lead dioxide and the negative plates consist of metallic lead which is gray and somewhat spongy. The electrolyte is about 33.5% sulfuric acid.

When the cell is discharging, the lead dioxide on the positive plate is converted into lead sulfate and water is formed. At the same time, at the negative plate, the lead there is also being converted to lead sulfate. When a lead-acid battery is completely discharged, both plates have become almost entirely converted to lead sulfate and the sulfuric acid solution has become mostly water. When both plates have changed to lead sulfate, they are chemically similar and no more current will flow.

Discharging reaction.

Negative plate reaction:

Pb(s) + HSO₄^-(aq) → PbSO₄(s) + H⁺(aq) + 2e^-

Positive plate reaction:

PbO₂(s) + HSO₄^-(aq) + 3H⁺(aq) + 2e^- → PbSO₄(s) + 2H₂O(l)

The total reaction can be written as

Pb(s) + PbO₂(s) + 2H₂SO₄(aq) → 2PbSO₄(s) + 2H₂O(l)

Charging reaction.

Negative plate reaction:

PbSO₄(s) + H⁺(aq) + 2e^- → Pb(s) + HSO₄^-(aq)

Positive plate reaction:

PbSO₄(s) + 2H₂O(l) → PbO₂(s) + HSO₄^-(aq) + 3H⁺(aq) + 2e^-

Effect of temperature on battery function. At low temperatures the open circuit emf of a lead-acid battery falls somewhat, as it does for all electrochemical reactions. The most important effect of low temperatures, however, is markedly increased internal resistance due to decreasing ion mobility within the battery. As a consequence, the terminal voltage drops abnormally when current is drawn. The cranking ability of an automobile storage battery at 0^o C is only about 40% of its value at 80^o C.

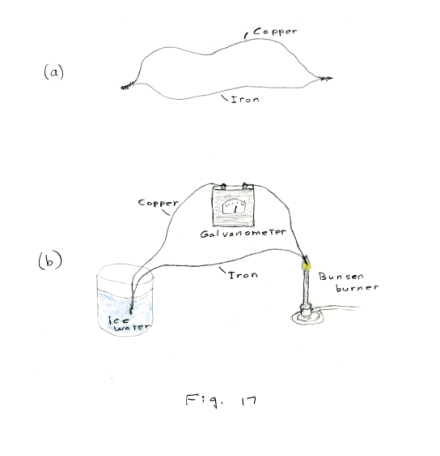
Thermoelectricity. Seebeck effect. Let us twist or solder together the ends of two different kinds of wire — say, iron and copper — to form a loop as shown in Fig. 17 (a). If, now, the junctions are maintained at different temperatures, a current will flow. If one places one junction of such a loop in ice water and the other junction in the flame of a Bunsen burner, as shown in Fig.17 (b), a galvanometer will show a current. This phenomenon is called the Seebeck effect after the German physicist Thomas J. Seebeck who discovered it in 1821. Such junctions are known as thermocouples and the emf in the circuit is called a thermal emf, or a Seebeck emf.

Let us label one of the junctions a reference junction, J_R, and the other junction a test junction, J_T.. If the temperature of the reference junction J_R is kept constant, as by keeping it immersed in ice water, the emf E_T at the test junction J_T is a function of the temperature T of the test junction. This fact enables the thermocouple to be used as a thermometer. The emf at the test junction is given, to a very close approximation, by

E_T = a + bT + cT²

where T is the temperature at the test junction and a, b, and c are constants for the particular thermocouple and have to be determined experimentally from measurements at known temperatures. Once these constants have been determined, the thermocouple can be used as a thermometer.

Because a thermocouple junction can be made very small, only very small quantities of heat are needed to produce a measurable effect. Due to its small size and mass, a thermocouple junction will follow very rapid changes in temperature.

Thermopile. The emf of a single thermocouple is very small. By connecting many thermocouples in series, one can construct an instrument called a thermopile. The total thermopile emf is the sum of the separate component thermocouple emf’s. Such a thermopile may register temperature changes of one one-hundred-millionth of a degree. Astronomers use such instruments to measure the heat of stars.

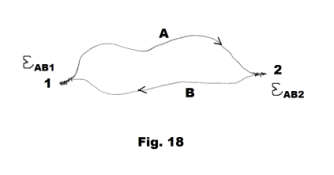
Explanation of the Seebeck effect: The Seebeck effect arises from the fact that the density of free electrons in a metal differs from one metal to another and, in a given metal, depends on temperature. When two different metals are connected to form a junction, electrons diffuse from one metal into the other. A consequence of this is that at any junction between two dissimilar metals, an emf E exists whose magnitude is a function of both the kind of metals forming the junction and the temperature. Consider the loop shown in Fig.18 constructed of two dissimilar metals A and B. We denote the junction emf at junction 1 by E_AB1 and the junction emf at junction 2 by E_AB2. Let us assume that there is a potential drop in going from metal A to metal B. Now the magnitudes of these junction potentials E_AB1 and E_AB2 are both dependent on the temperature at the joints. If the temperature at both junctions is the same, these junction emf’s will be of equal magnitude and will oppose each other and no current will flow. If the temperature at junction 2 is greater than that at junction 1, E_AB2 will be greater than E_AB1 and current will flow in the clockwise direction. If the temperature at junction 1 is greater than that at junction 2, E_AB1 will be greater than E_AB2 and current will flow in the counterclockwise direction.

There are two other thermoelectric effects closely connected to the Seebeck effect: the Peltier effect and the Thomson effect.

1. Peltier effect. When a direct current is passed through a junction formed by two dissimilar metals, the junction becomes warmer or cooler depending on the direction of the current. When the current is in the direction of the junction emf, the temperature of the junction falls, while if it is in the opposite direction, the temperature of the junction rises.

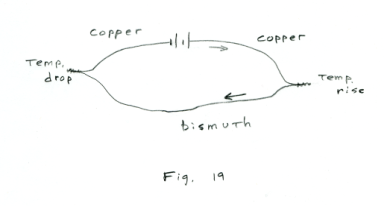
Example. In a circuit consisting of a battery joined by two pieces of copper wire to a length of bismuth wire, a temperature rise occurs at the junction where the current passes from copper to bismuth, and a temperature drop occurs at the junction where the current passes from bismuth to copper. See Fig. 19.

The heat that is given off or absorbed at a junction is known as Peltier heat, after its discoverer, Jean C. A. Peltier, a French physicist. Experiment has shown that the amount of Peltier heat absorbed or liberated at any junction is proportional to the quantity of electricity crossing the junction.

Def. Peltier emf, π_AB. The number of joules of heat absorbed or liberated at a junction of metals A and B per coulomb of electricity transferred:

The Peltier emf π_AB depends on the nature of the two metals and on the temperature at the junction and has been found to be independent of any other junction that may be present.

2. Thomson effect. In a wire in which the temperature varies from point to point (as for example in one whose ends are maintained at different temperatures), the free electron density varies from point to point with the temperature (the free electron density is highest at points where the temperature is lowest). As a consequence of this, differences of potential may be observed along the wire corresponding to the varying free electron density. Each infinitesimal element of a wire of nonuniform temperature is thus a seat of emf, a discovery made by Sir William Thomson (Lord Kelvin). When a current is maintained in a wire of nonuniform temperature, heat is liberated or absorbed at all points of the wire. This heat, called Thomson heat, is proportional to the quantity of electricity passing the section of wire and to the temperature difference between the ends of the section.

Def. Thomson emf, σ_Adt. If an infinitesimal length of wire A has a temperature difference dt (i.e. dt is temperature difference between the beginning and end of the section), the number of joules of heat absorbed or liberated in this length of wire per coulomb of electricity transferred is called the Thomson emf, σ_Adt

The total Thomson emf in a wire whose ends are at temperatures t₁ and t₂ is

The coefficient σ_A is sometimes called the “specific heat of electricity”.

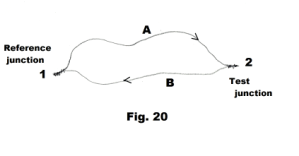
Fundamental thermocouple equation relating the Seebeck effect, Peltier effect and Thomson effect. Let junction 1 in Fig. 20 be the reference junction, junction 2 be the test junction, t_R be the temperature of the reference junction, and t be the temperature of the test junction. Then the Seebeck emf E_AB is the sum of the emf’s around the loop:

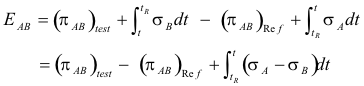
The sign conventions for this equation are:

1. E_AB is positive when the direction of the thermocouple current is from A to B at the test junction, where the test junction is taken to be the warmer of the two junctions.

2. π_AB is positive when the current is from A to B and Peltier heat is absorbed by the junction.

3. σ_A is positive when the current is opposite to the direction of the temperature gradient (low to high) and Thomson heat is absorbed.

References

1. Dull, Metcalfe, Brooks. Modern Physics.

2. Sears, Zemansky. University Physics

3. Semat, Katz. Physics.

4. World Book encyclopedia

5. Dull, Metcalfe, Williams. Modern Physics.

6. Wikipedia